
Evaluating Definite Integrals. This power point presentation is created and written by Dr. Julia Arnold Using the 5 th edition of S. T. Tan’s text Applied Calculus. Properties of the Definite Integral. Rule 1:. Rule 2:. Rule 3: c, a constant. Rule 4:.

lefty + Follow
Download PresentationAn Image/Link below is provided (as is) to download presentation Download Policy: Content on the Website is provided to you AS IS for your information and personal use and may not be sold / licensed / shared on other websites without getting consent from its author. Content is provided to you AS IS for your information and personal use only. Download presentation by click this link. While downloading, if for some reason you are not able to download a presentation, the publisher may have deleted the file from their server. During download, if you can't get a presentation, the file might be deleted by the publisher.

5.2 Definite Integrals. Greg Kelly, Hanford High School, Richland, Washington. When we find the area under a curve by adding rectangles, the answer is called a Rieman sum . The width of a rectangle is called a subinterval . The entire interval is called the partition . subinterval.
454 views • 19 slides

Definite Integrals. As taught by Nathan "Definite" Shagam and Ethan "Integral" Scholl.
691 views • 14 slides

5.2 Definite Integrals. Sigma Notation. What does the following notation mean?. means the sum of the numbers from the lower number to the top number. Area under curves. In 5.1, we found that we can approximate areas using rectangles. How do we get a more accurate approximation for area?.
359 views • 12 slides

Applications of Definite Integrals. Section 8.2. Areas in the Plane. Area between the curve and x-axis. Area Between Curves. Area Between Curves. Example 1 – Using the definition. Example 1. Example 2. Example 3 Area of an Enclosed Region. Example 3 Area of an Enclosed Region.
707 views • 17 slides

4-3 definite integrals. Ms. Battaglia – ap calculus . Definite integral. A definite integral is an integral with upper and lower bounds. The number a is the lower limit of integration, and the number b is the upper limit of integration.
718 views • 14 slides
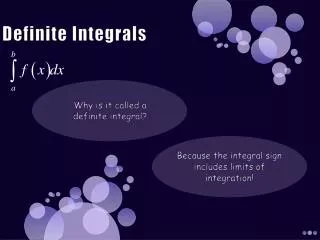
Definite Integrals. Why is it called a definite integral?. Because the integral sign includes limits of integration!. Properties of Definite Integrals. Properties of Definite Integrals. Properties of Definite Integrals. Why?
593 views • 6 slides

Definite Integrals. 5.6 “u” substitution. The First Fundamental Theorem of Calculus. To understand this, lets do a problem in 2 steps: Find F(x): Find F’(x) . The First Fundamental Theorem of Calculus. If f is continuous on [a,b] then is differentiable on
224 views • 8 slides

5.2 Definite Integrals. Gre Kelly, Hanford High School, Richland, Washington. When we find the area under a curve by adding rectangles, the answer is called a Rieman sum. The width of a rectangle is called a subinterval. The entire interval is called the partition. subinterval.
394 views • 27 slides

Areas and Definite Integrals. Objectives. Students will be able to Calculate a definite integral. Calculate the area between a curve and the x -axis over a given interval. Use definite integrals to application problems. Fundamental Theorem of Calculus.
384 views • 11 slides

5.2 Definite Integrals. When we find the area under a curve by adding rectangles, the answer is called a Riemann sum. The width of a rectangle is called a subinterval. The entire interval is called the partition. subinterval. partition. Subintervals do not all have to be the same size.
340 views • 21 slides

5.2 Definite Integrals. Greg Kelly, Hanford High School, Richland, Washington. When we find the area under a curve by adding rectangles, the answer is called a Rieman sum. The width of a rectangle is called a subinterval. The entire interval is called the partition. subinterval.
290 views • 19 slides

5.2 Definite Integrals. Greg Kelly, Hanford High School, Richland, Washington. Quick Review. What you’ll learn about. Riemann Sums The Definite Integral Computing Definite Integrals on a Calculator Integrability … and why The definite integral is the basis of integral calculus,
331 views • 14 slides

5.2 Definite Integrals. Greg Kelly, Hanford High School, Richland, Washington. Definite Integrals. 5.2. Riemann Sums The Definite Integral Computing Definite Integrals on a Calculator Integrability … and why The definite integral is the basis of integral calculus,
529 views • 18 slides
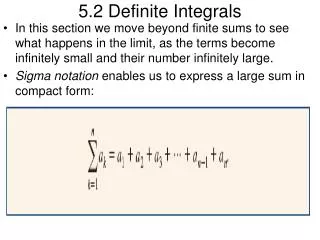
5.2 Definite Integrals. In this section we move beyond finite sums to see what happens in the limit, as the terms become infinitely small and their number infinitely large. Sigma notation enables us to express a large sum in compact form:. Definite Integrals.
561 views • 20 slides
 Integrals by Substitution" width="320px" />
Integrals by Substitution" width="320px" />
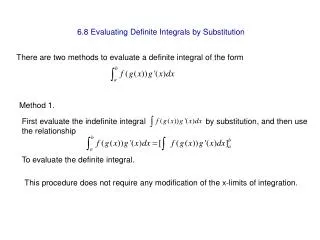
6.8 Evaluating Definite Integrals by Substitution. There are two methods to evaluate a definite integral of the form. Method 1. First evaluate the indefinite integral by substitution, and then use the relationship To evaluate the definite integral.
350 views • 8 slides

Definite Integrals. Riemann Sums and Trapezoidal Rule. Why all this work? Why learn integration?. We are now going to look to find the numerical value of any curve f(x) from a to b, bounded by the x axis. (Fig 1)
329 views • 9 slides

Application of Definite Integrals. Dr. Farhana Shaheen Assistant Professor YUC- Women Campus. Calculus ( Latin , calculus , a small stone used for counting).
1.11k views • 65 slides

5.2 Definite Integrals. When we find the area under a curve by adding rectangles, the answer is called a Riemann sum. The width of a rectangle is called a subinterval.
152 views • 12 slides

Definite Integrals. 6.2. Summation Notation. When we find the area under a curve by adding rectangles, the answer is called a Riemann sum. The width of a rectangle is called a subinterval. The entire interval is called the partition. subinterval. partition.
388 views • 34 slides

Properties of Definite Integrals. Objectives. Students will be able to Calculate a definite integral using the properties of definite integrals. Calculate the area between a curve and the x -axis over a given interval. Use definite integrals to application problems.
212 views • 9 slides

5.2 Definite Integrals. Greg Kelly, Hanford High School, Richland, Washington Adapted by: Jon Bannon , Siena College. When we find the area under a curve by adding rectangles, the answer is called a Rieman sum. The width of a rectangle is called a subinterval.
156 views • 14 slides

5.2 Definite Integrals. Greg Kelly, Hanford High School, Richland, Washington. When we find the area under a curve by adding rectangles, the answer is called a Rieman sum. The width of a rectangle is called a subinterval. The entire interval is called the partition. subinterval.
235 views • 19 slides